Article Sidebar

Download
PDF
Statistic
Read Counter : 67 Download : 56

Downloads

Download data is not yet available.

Main Article Content

Abstract

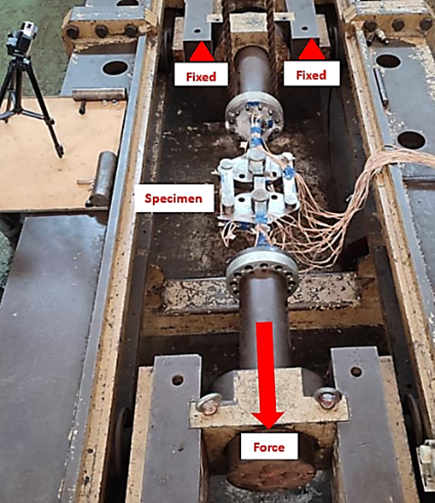
Topology optimization has demonstrated its effectiveness in generating lightweight and structurally efficient designs. This study focuses on refining the geometry of an automatic coupler body for trains using solid isotropic material with penalization and a level set method. These optimization methods are applied to the numerical model of the automatic coupler, and their results are compared to select the optimal design. The tensile strength of the automatic coupler is examined through numerical simulations and validated by experimental tensile tests conducted on a 1:1 scale prototype. The optimization outcomes reveal a remarkable 46.41% reduction in the mass of the automatic coupler body compared to the initial model. An evaluation of the tensile strength of the prototype demonstrates the ability of the automatic coupler to withstand the primary load without undergoing plastic deformation. Furthermore, a strong correlation is observed between the numerical and experimental results. This research contributes to advancing the design of next-generation automatic couplers, emphasizing the crucial aspects of lightweight design and structural performance.

Keywords

Topology optimization Automatic coupler Lightweight design Tensile test Verification and validation

Article Details

Creative Commons License

This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.

References

  1. Y. Lei, “Lightweight Design of High-speed Train under the Development of New Materials,” in 5th International Conference on Vehicle, Mechanical and Electrical Engineering, 2020, pp. 217–221, doi: 10.5220/0008865102170221.
  2. E. de la Guerra, “Lightweight primary structures for High-speed railway carbodies,” International Congress on High-speed Rail: Technologies and Long Term Impacts, vol. 5, pp. 1-9–21, 2018.
  3. D. W. Karmiadji, B. Haryanto, O. Ivano, M. Perkasa, and A. R. Farid, “Bogie Frame Structure Evaluation for Light-Rail Transit (LRT) Train: A Static Testing,” Automotive Experiences, vol. 4, no. 1, pp. 36–43, 2021, doi: 10.31603/ae.4252.
  4. D. W. Karmiadji, M. Gozali, A. Anwar, H. Purnomo, M. Setiyo, and R. Junid, “Evaluation of Operational Loading of the Light-Rail Transit (LRT) in Capital Region, Indonesia,” Automotive Experiences, vol. 3, no. 3, pp. 104–114, 2020, doi: 10.31603/ae.v3i3.3882.
  5. A. Cascino, E. Meli, and A. Rindi, “A strategy for lightweight designing of a railway vehicle car body including composite material and dynamic structural optimization,” Railway Engineering Science, vol. 31, no. 4, pp. 340–350, Dec. 2023, doi: 10.1007/s40534-023-00312-6.
  6. M. Seitzberger, R. Nedelik, A. Ruthmeier, and T. Grausgruber, “CAE-based concept development for lightweight design of railway vehicles,” Lightweight Design worldwide, vol. 10, no. 5, pp. 32–37, Oct. 2017, doi: 10.1007/s41777-017-0044-y.
  7. M. Watson, M. Leary, and M. Brandt, “Generative design of truss systems by the integration of topology and shape optimisation,” The International Journal of Advanced Manufacturing Technology, vol. 118, no. 3–4, pp. 1165–1182, Jan. 2022, doi: 10.1007/s00170-021-07943-1.
  8. H. Sun and L. Ma, “Generative Design by Using Exploration Approaches of Reinforcement Learning in Density-Based Structural Topology Optimization,” Designs, vol. 4, no. 2, p. 10, May 2020, doi: 10.3390/designs4020010.
  9. S. Jang, S. Yoo, and N. Kang, “Generative Design by Reinforcement Learning: Enhancing the Diversity of Topology Optimization Designs,” Computer-Aided Design, vol. 146, p. 103225, May 2022, doi: 10.1016/j.cad.2022.103225.
  10. S. Johnsen, “Structural Topology Optimization: Basic Theory, Methods and Applications,” Norwegian University of Science and Technology, 2013.
  11. J. Gao, M. Xiao, Y. Zhang, and L. Gao, “A Comprehensive Review of Isogeometric Topology Optimization: Methods, Applications and Prospects,” Chinese Journal of Mechanical Engineering, vol. 33, no. 1, p. 87, Dec. 2020, doi: 10.1186/s10033-020-00503-w.
  12. P. W. Christensen and A. Klarbring, An Introduction to Structural Optimization. Springer, 2008.
  13. P. K. Srivastava, “Reducing Weight of Freight Bogie Bolster Using Topology Optimization,” Revista Gestão Inovação e Tecnologias, vol. 11, no. 3, pp. 324–339, Jun. 2021, doi: 10.47059/revistageintec.v11i3.1941.
  14. T. Ide, M. Otomori, J. P. Leiva, and B. C. Watson, “Structural optimization methods and techniques to design light and efficient automatic transmission of vehicles with low radiated noise,” Structural and Multidisciplinary Optimization, vol. 50, no. 6, pp. 1137–1150, Dec. 2014, doi: 10.1007/s00158-014-1143-6.
  15. E. Tyflopoulos, M. Lien, and M. Steinert, “Optimization of Brake Calipers Using Topology Optimization for Additive Manufacturing,” Applied Sciences, vol. 11, no. 4, p. 1437, Feb. 2021, doi: 10.3390/app11041437.
  16. M. Mrzygłód and T. Kuczek, “Uniform crashworthiness optimization of car body for high-speed trains,” Structural and Multidisciplinary Optimization, vol. 49, no. 2, pp. 327–336, Feb. 2014, doi: 10.1007/s00158-013-0972-z.
  17. N. M. Patel, B.-S. Kang, J. E. Renaud, and A. Tovar, “Crashworthiness Design Using Topology Optimization,” Journal of Mechanical Design, vol. 131, no. 6, Jun. 2009, doi: 10.1115/1.3116256.
  18. J. M. Valentino, A. S. Pramono, and A. Syaifudin, “Design Optimization of Hooked Plate on the Automatic Coupler for High-Speed Train,” in Recent Advances in Mechanical Engineering, 2023, pp. 10–18.
  19. ASME, “Guide for Verification and Validation in Computational Solid Mechanics,” American Society of Mechanical Engineers, no. March, pp. 1–53, 2006.
  20. J. Mario, A. Syaifudin, A. S. Pramono, A. Windharto, and A. Farid, “Synthesis Analysis to Improve Coupling Strength of Automatic Coupler,” Mar. 2023, pp. 67–73, doi: 10.4028/p-higyh1.
  21. M. P. Bendsøe and O. Sigmund, Topology Optimization: Theory, Methods, and Applications. Berlin, Heidelberg: Springer Berlin Heidelberg, 2004.
  22. M. Abdi, “Evolutionary topology optimization of continuum structures using X-FEM and isovalues of structural performance,” University of Nottingham, 2015.
  23. P. Wei, W. Wang, Y. Yang, and M. Y. Wang, “Level set band method: A combination of density-based and level set methods for the topology optimization of continuums,” Frontiers of Mechanical Engineering, vol. 15, no. 3, pp. 390–405, Sep. 2020, doi: 10.1007/s11465-020-0588-0.
  24. W. Zuo and K. Saitou, “Multi-material topology optimization using ordered SIMP interpolation,” Structural and Multidisciplinary Optimization, vol. 55, no. 2, pp. 477–491, Feb. 2017, doi: 10.1007/s00158-016-1513-3.
  25. R. Picelli, S. Townsend, C. Brampton, J. Norato, and H. A. Kim, “Stress-based shape and topology optimization with the level set method,” Computer Methods in Applied Mechanics and Engineering, vol. 329, pp. 1–23, Feb. 2018, doi: 10.1016/j.cma.2017.09.001.
  26. S. Liu, Q. Li, J. Liu, W. Chen, and Y. Zhang, “A Realization Method for Transforming a Topology Optimization Design into Additive Manufacturing Structures,” Engineering, vol. 4, no. 2, pp. 277–285, Apr. 2018, doi: 10.1016/j.eng.2017.09.002.
  27. M. Noda, K. Matsushima, and T. Yamada, “Orientation optimization via topological derivatives in combination with multi-material topology optimization based on extended level set method,” Computer Methods in Applied Mechanics and Engineering, vol. 418, p. 116585, Jan. 2024, doi: 10.1016/j.cma.2023.116585.
  28. ASTM, “ASTM E8 Standard Test Methods for Tension Testing of Metallic Materials.” ASTM International, Conshohocken, 2013, [Online]. Available: https://www.astm.org/DATABASE.CART/HISTORICAL/E8E8M-13.htm.
  29. J. Valentino et al., “Enhancing S45C steel for the primary component of an automatic coupler using quench-tempering techniques,” Journal of Applied Engineering Science, vol. 22, no. 2, pp. 215–222, 2024, doi: 10.5937/jaes0-43988.
  30. P. Arasaratnam, K. S. Sivakumaran, and M. J. Tait, “True Stress-True Strain Models for Structural Steel Elements,” ISRN Civil Engineering, vol. 2011, pp. 1–11, Aug. 2011, doi: 10.5402/2011/656401.
  31. M. Jeyakumar and T. Christopher, “Influence of residual stresses on failure pressure of cylindrical pressure vessels,” Chinese Journal of Aeronautics, vol. 26, no. 6, pp. 1415–1421, Dec. 2013, doi: 10.1016/j.cja.2013.07.025.
  32. Y. L. Yap et al., “Topology optimization and 3D printing of micro-drone: Numerical design with experimental testing,” International Journal of Mechanical Sciences, vol. 237, p. 107771, Jan. 2023, doi: 10.1016/j.ijmecsci.2022.107771.
  33. B. Xie, X. Wu, L. Liu, and Y. Zhang, “Topological Design of a Hinger Bracket Based on Additive Manufacturing,” Materials, vol. 16, no. 11, p. 4061, May 2023, doi: 10.3390/ma16114061.
  34. P. K. Sahoo, S. Pattnaik, and M. K. Sutar, “A State-of-the-Art Review on Manufacturing and Additive Influences on Sand-Cast Components,” Arabian Journal for Science and Engineering, vol. 44, no. 12, pp. 9805–9835, Dec. 2019, doi: 10.1007/s13369-019-04139-4.
  35. M. Maass, W. A. Deutsch, and F. Bartholomai, “Magnetic Particle Inspection on train components,” 2014.
  36. M. Gupta, M. A. Khan, R. Butola, and R. M. Singari, “Advances in applications of Non-Destructive Testing (NDT): A review,” Advances in Materials and Processing Technologies, vol. 8, no. 2, pp. 2286–2307, Apr. 2022, doi: 10.1080/2374068X.2021.1909332.
  37. P. Tutak, “Application Of Strain Gauges In Measurements Of Strain Distribution In Complex Objects,” Journal of Applied Computer Science Methods, vol. 6, no. 2, pp. 135–145, Dec. 2014, doi: 10.1515/jacsm-2015-0004.
  38. J. Arpin-Pont, M. Gagnon, A. S. Tahan, A. Coutu, and D. Thibault, “Methodology for estimating strain gauge measurement biases and uncertainties on isotropic materials,” The Journal of Strain Analysis for Engineering Design, vol. 50, no. 1, pp. 40–50, Jan. 2015, doi: 10.1177/0309324714550116.

Most read articles by the same author(s)